
We consider trajectories in the configuration space of a freely rotating rigid body, the center-of-mass of which is held fixed. A point in this space corresponds to a angular position which is most conveniently described by a triplet of Euler-Rodrigues parameters. Initial conditions for trajectories specify angular position r0 and angular velocity ω0. During a short span of time Δt a trajectory can be approximated by a parabolic trajectory which can be defined in a natural manner by supplementing the initial conditions with a single constant vector α of angular accelertation. A working method for computing trajectories for given initial conditions is by means of an algorithm which gives just this angular acceleration as a function of initial angular position, initial angular velocity, and time step. This defines an integrator which can be used for building up a time-discrete trajectory. Here we study a specific algorithm of this kind which is given by equation (52) in Ulrich Mutze: Rigidely connected overlapping spherical particles: a versatile grain model, Granular Matter (2006) or equation (59) in article8 . We have α=Δω/Δt where the quantities on the r.h.s. are those in equation (52). The animation shows that this formula defines trajectories which approximately satisfy the principle of least action. We are now in a position to explain what precisely is shown in this animation.
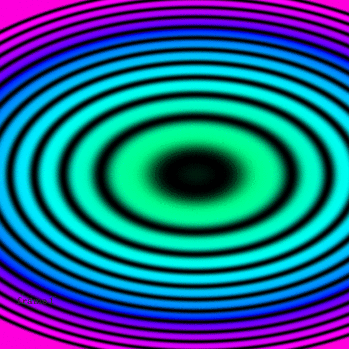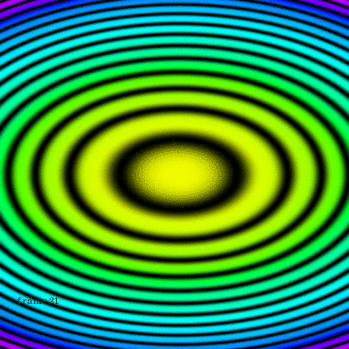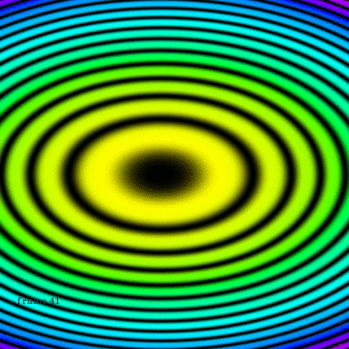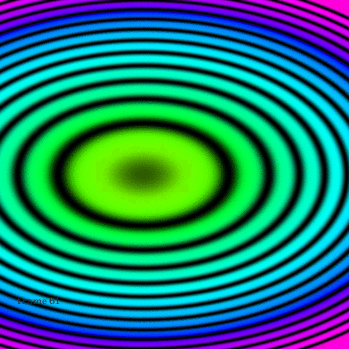
Each pixel of each frame corresponds to a finite parabolic trajectory and the pixel color corresponds to the value of the action integral along this trajectory. Violet colors correspond to the largest values, red colors to the lowest ones ('photon energy color coding'). The dark bands mark numerical values just like Fraunhofer lines mark frequencies in the solar spectrum. They form lines of equal action. They give a good indication where the action takes a minimum.
All parabolic paths under consideration are variations of an approximate physical trajectory p which is taken as originating from rather arbitrary initial conditions r and ω by one time-step of duration Δt of the integrator mentioned above. The varied trajectories coincide with p for t = 0 and t = Δt. Each such varied trajectory is characterized by an acceleration vector α + dα, where α is the acceleration vector of p. The action integral along the trajectory characterized by acceleration α + dα (thus having initial velocity ω - ½Δtdα ) should therefore have a minimum near dα = 0 as a result of Hamilton's principle of least action. To visualize the action as a function of the 3-dimensional quantity dα, we decompose it in 3 real components: one in the direction of ω and two perpendicular to ω. The longitudinal component determines the number of the frame in which the corresponding action values get represented (value 0 of this component corresponds to the mid-frame, which is frame # 31 ). The two transversal components determine the coordinates within a frame. The y-direction of the frame corresponds to the initial direction of the principal axis associated with the largest moment of inertia. Actually each of the 61 frames was computed from 81 times 81 data points by interpolation, so that the action along 400221 paths had to be computed. All visible noise and and artifacts are due to the conversion of the original ppm-images to a single gif-image. One sees that the actual minimum is reached at a slightly different position (frame # 34, slightly left to the center) than the one corresponding to dα = 0 (frame # 31, at the center). Notice that expanding rings indicate that the minimum becomes deeper and that contracting rings indicate that the value at the minimum increases.
These are the data adopted for the computation: The moments of inertia are (1.0, 1.4142, 3). The initial angular position has Euler-Rodrigues parameters (1,1,1) with respect to the axes to which these momenta of inertia refer. The initial angular velocity is (0,5,10) and Δt is 0.0002. For these data, the integrator (52) gives the angular acceleration as α = (-6.9033, -0.0207, 0.0073). The absolute value |α| of α sets the scale for the representation of dα: the frames cover all values da the components of which are less than 10% of |α| in absolute value. UM 2006-06-26, last change 2008-05-01